Rate of Turn

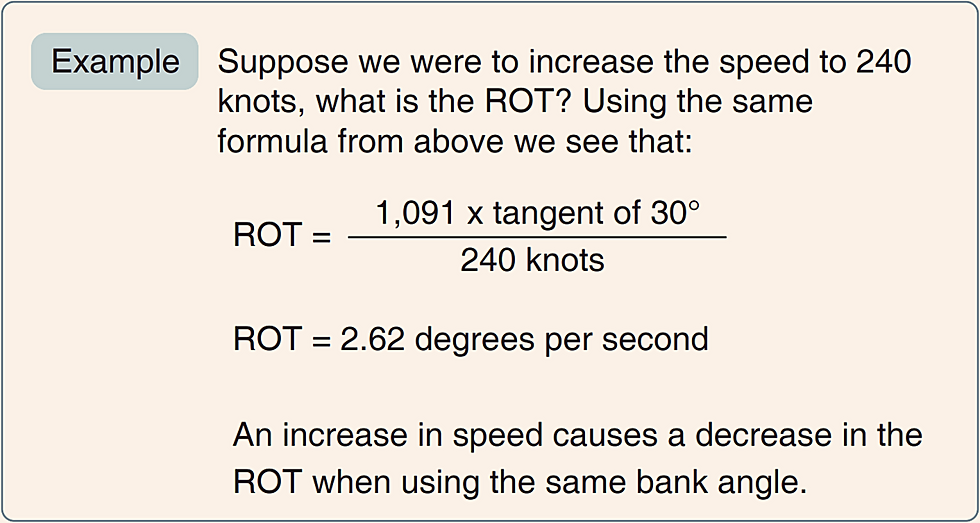
The rate of turn (ROT) is the number of degrees (expressed in degrees per second) of heading change that an aircraft makes. The ROT can be determined by taking the constant of 1,091, multiplying it by the tangent of any bank angle and dividing that product by a given airspeed in knots as illustrated in Figure 5-56. If the airspeed is increased and the ROT desired is to be constant, the angle of bank must be increased, otherwise, the ROT decreases. Likewise, if the airspeed is held constant, an aircraft’s ROT increases if the bank angle is increased. The formula in Figures 5-56 through 5-58 depicts the relationship between bank angle and airspeed as they affect the ROT.

NOTE: All airspeed discussed in this section is true airspeed (TAS).

[Figure 5-56]

[Figure 5-57]

[Figure 5-58]

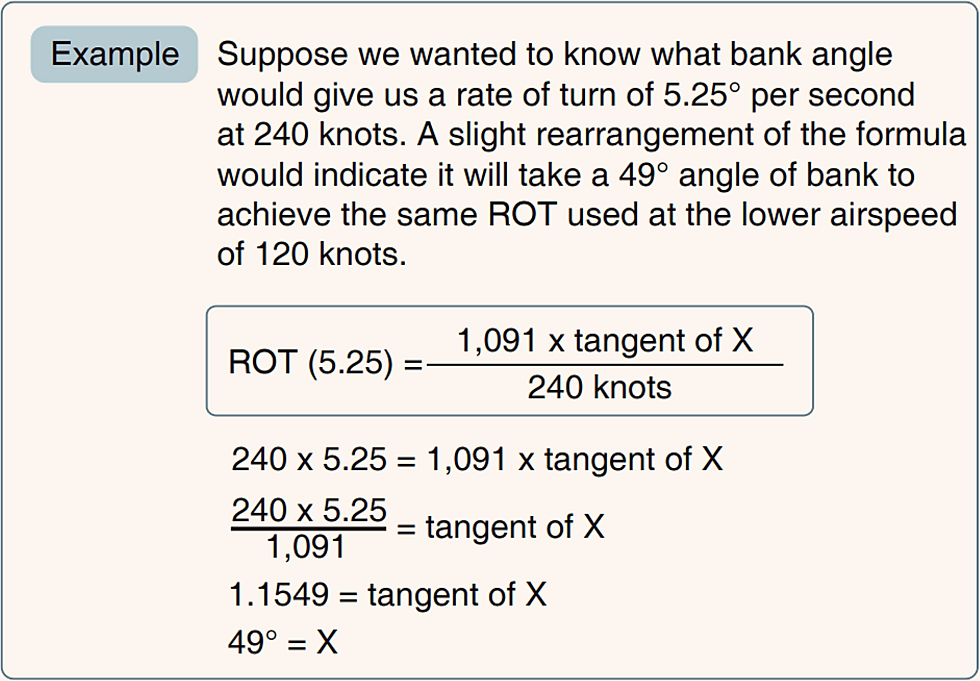
Airspeed significantly effects an aircraft’s ROT. If airspeed is increased, the ROT is reduced if using the same angle of bank used at the lower speed. Therefore, if airspeed is increased as illustrated in Figure 5-57, it can be inferred that the angle of bank must be increased in order to achieve the same ROT achieved in Figure 5-58.

What does this mean on a practicable side? If a given airspeed and bank angle produces a specific ROT, additional conclusions can be made. Knowing the ROT is a given number of degrees of change per second, the number of seconds it takes to travel 360° (a circle) can be determined by simple division. For example, if moving at 120 knots with a 30° bank angle, the ROT is 5.25° per second and it takes 68.6 seconds (360° divided by 5.25 = 68.6 seconds) to make a complete circle. Likewise, if flying at 240 knots TAS and using a 30° angle of bank, the ROT is only about 2.63° per second and it takes about 137 seconds to complete a 360° circle. Looking at the formula, any increase in airspeed is directly proportional to the time the aircraft takes to travel an arc.

So why is this important to understand? Once the ROT is understood, a pilot can determine the distance required to make that particular turn, which is explained in radius of turn.

Radius of Turn

The radius of turn is directly linked to the ROT, which explained earlier is a function of both bank angle and airspeed. If the bank angle is held constant and the airspeed is increased, the radius of the turn changes (increases). A higher airspeed causes the aircraft to travel through a longer arc due to a greater speed. An aircraft traveling at 120 knots is able to turn a 360° circle in a tighter radius than an aircraft traveling at 240 knots. In order to compensate for the increase in airspeed, the bank angle would need to be increased.

The radius of turn (R) can be computed using a simple formula. The radius of turn is equal to the velocity squared (V2) divided by 11.26 times the tangent of the bank angle.

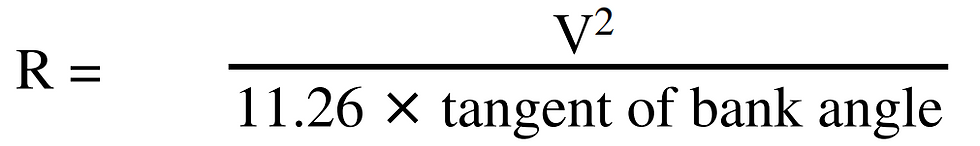
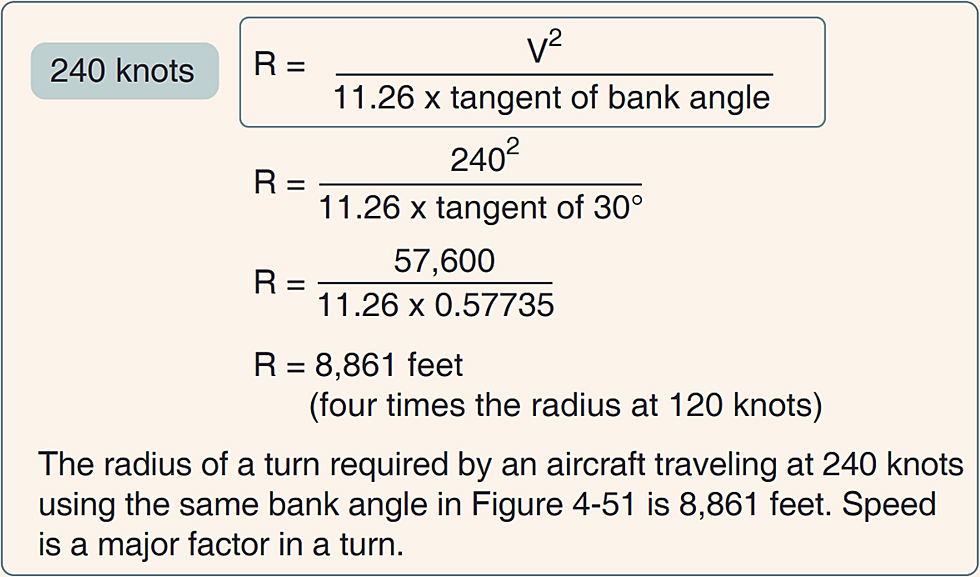
Using the examples provided in Figures 5-56 through 5-58, the turn radius for each of the two speeds can be computed.

Note that if the speed is doubled, the radius is quadrupled. [Figures 5-59 and 5-60]

[Figures 5-59]

[Figures 5-60]

Another way to determine the radius of turn is speed using feet per second (fps), π (3.1415), and the ROT. In one of the previous examples, it was determined that an aircraft with a ROT of 5.25 degrees per second required 68.6 seconds to make a complete circle. An aircraft’s speed (in knots) can be converted to fps by multiplying it by a constant of 1.69. Therefore, an aircraft traveling at 120 knots (TAS) travels at 202.8 fps. Knowing the speed in fps (202.8) multiplied by the time an aircraft takes to complete a circle (68.6 seconds) can determine the size of the circle; 202.8 times 68.6 equals 13,912 feet. Dividing by π yields a diameter of 4,428 feet, which when divided by 2 equals a radius of 2,214 feet [Figure 5-61], a foot within that determined through use of the formula in Figure 5-59.

[Figures 5-61]

In Figure 5-62, the pilot enters a canyon and decides to turn 180° to exit. The pilot uses a 30° bank angle in his turn.

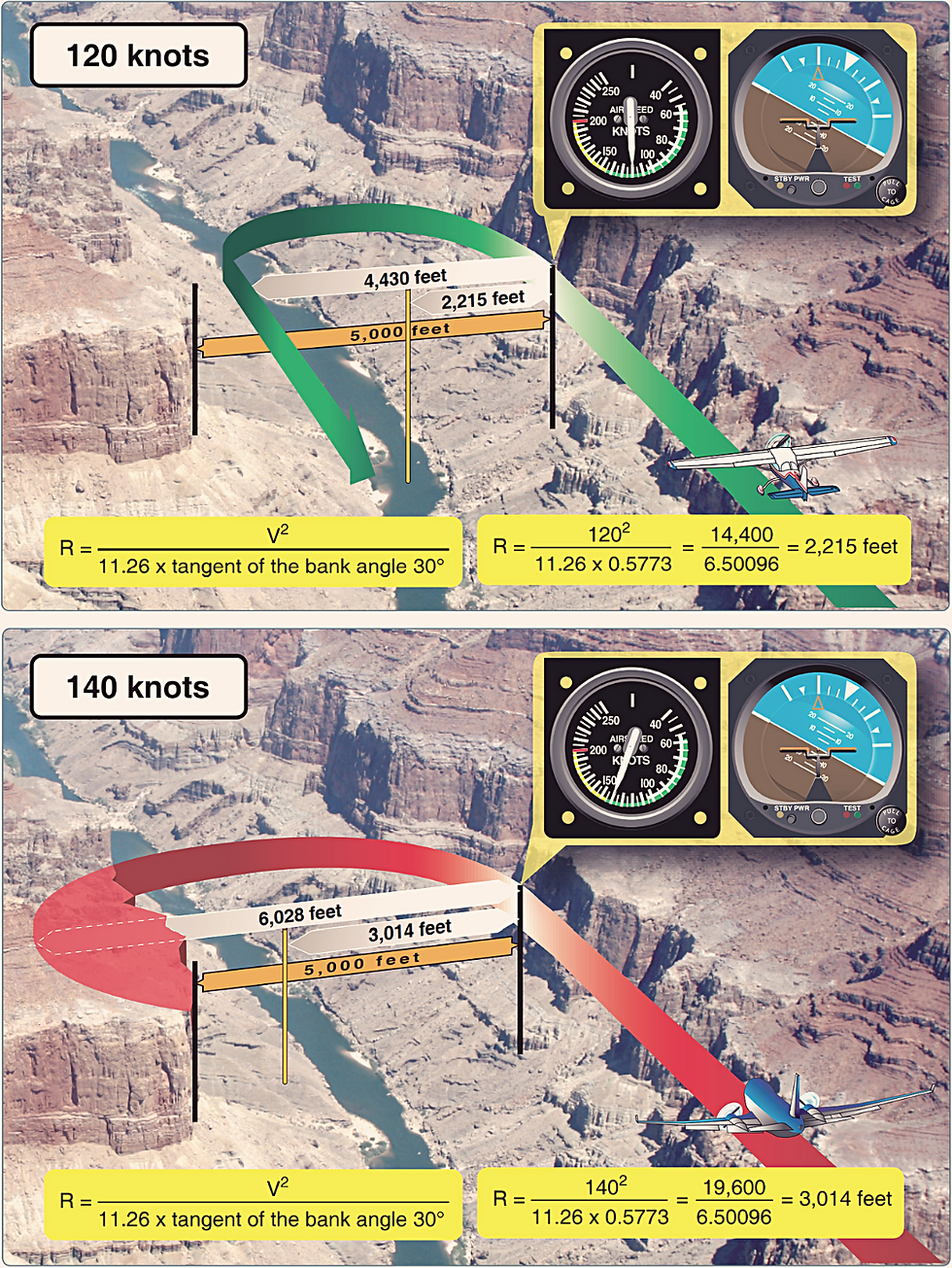
[Figures 5-62]

Next

Return to Performance and Limitations

Back

Become a Pilot

Still Growing

This website is in the very early stages of development at the moment. While I'm currently working on pulling information from the Pilot’s Handbook of Aeronautical Knowledge my next goal will be to process information from all the documents referenced in the private pilot’s Airman Certification Standards. So, please come back as I'll regularly be providing you with more and more information.